Between 1900 and 1905, the Wright brothers designed and built three
unpowered gliders and three powered aircraft.
In the design of each aircraft, the brothers tried to
maximize the
lift to drag ratio because
high
lift
and low
drag
gives the best airplane
performance.
How did they predict the lift and drag of their design?
The Wright brothers were bicycle mechanics and designers
and they had a good working knowledge of math and science. They knew about
Newton's laws
of motion and about
forces
and
torques.
They had written to the Smithsonian when they began their enterprise in 1899
and received technical papers describing the aeronautical
theories of the day. There were mathematical equations which could
be used to predict the amount of
lift
and drag
that an object would generate. The
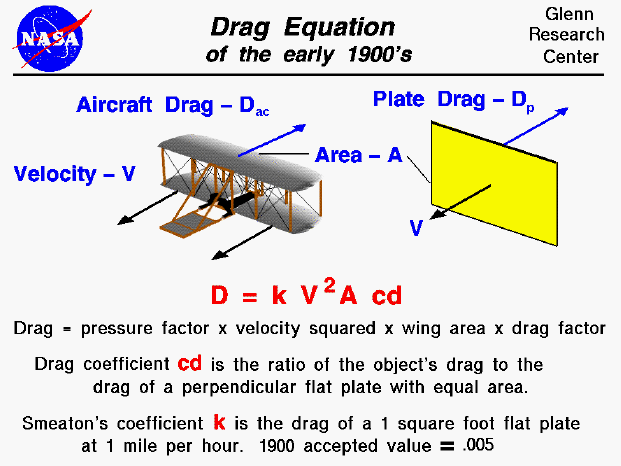
drag equation is shown on this slide.
The amount of drag generated by an object depends on a number of factors,
including
properties
of the air, the
velocity between the object and the
air, the surface area
over which the air flows, the
shape
of the body, and the body's inclination to the flow, also called the
angle of attack.
By the time the Wrights began their studies, it had been determined that
drag depends on the
square of the velocity
and varies linearly
with the surface area of the object.
Early aerodynamicists characterized the dependence on the properties of the air
by a pressure coefficient called
Smeaton's coefficient which represented the
pressure force (drag) on a one foot square flat plate moving at one mile per hour through
the air. They believed that any object moving through the air converted some
portion of the pressure force into drag, and they represented that portion by a
drag coefficient. The resulting equation is given as:
D = k * V^2 * A * cd
where D is the drag, k is the Smeaton coefficient, V is the velocity,
A is the wing area, and cd is the drag coefficient.
This equation is slightly different from the modern
drag equation
used today. The modern equation uses the
dynamic pressure
of the moving air for the pressure dependence, while this equation uses
the Smeaton coefficient. Modern drag coefficients relate the drag force on the object to
the force generated by the dynamic pressure times the area, while the 1900's
drag coefficients relate the drag force to the drag of a flat plate of equal area.
For the modern drag equation the drag coefficient of a flat plate
moving perpendicular to the flow is 1.28; for the 1900's drag equation,
the drag coefficient for this problem is
equal to 1.0.
The 1900's equation assumes that you know the perpendicular pressure force on a
moving flat plate (Smeaton coefficient). Because
of measuring inaccuracies at the time, there were many quoted values for the
coefficient ranging from .0027 to .005. Lilienthal had used the .005 value
in the design and testing of his wings.
When the Wrights began to design the 1900 aircraft,
they used values for the drag coefficient based on the work by Lilienthal
so they too used the .005 value.
During the kite and glider
experiments of 1900 and 1901, the brothers measured the performance
of their aircraft. Neither aircraft performed as well as predicted
by the lift and drag equations. The 1901 aircraft
had been designed to lift itself (100 pounds) plus a pilot (150 pounds)
when flown as a kite in a 15 mile per hour wind at 5 degrees angle of attack.
But in flight, it could barely lift itself in a 15 mile per hour wind at
a much higher angle of attack.
So the brothers began to doubt the .005 value for the Smeaton coefficient and
they determined that a value of .0033 more closely approximated their data.
The modern accepted value is .00326.
The brothers also began to doubt the accuracy of Lilienthal's lift and drag
coefficients.
So in the fall of 1901, they decided to determine their own values
for the drag coefficient using a
wind tunnel.
The brothers built a clever
balance
to directly measure the ratio of the drag of their models to the
lift of the model. They built another
balance
to determine the lift.
We have developed an
interactive tunnel simulator
so that you can duplicate their wind tunnel results.
In the
process
of testing many airfoil models, the brothers discovered the importance
of wing
shape
on the drag coefficient.
They determined that the Lilienthal data was correct for the wing geometry
that he had used, but that the data could not be applied to a wing with a very
different geometry. Lilienthal's wings had a rather short span and an elliptical
planform, while the brothers used a long, thin, rectangular planform.
The brothers tested over fifty different
models
to determine
how lift and drag are affected by various design parameters
and they used this data to design their 1902 aircraft
using the drag equation shown on the slide with their own drag coefficients.
The brothers determined the power requirements for the 1903 aircraft
based on their drag data.
You can view a short
movie
of "Orville and Wilbur Wright" discussing the drag force
and how it affected the flight of their aircraft. The movie file can
be saved to your computer and viewed as a Podcast on your podcast player.
Navigation..



- Beginner's Guide Home Page
|
