Knots Versus Miles per Hour

Knots is how the speed of aircraft and boats is measured. Both miles per hour and knots is a speed which is the number of units of distance that is covered for a certain amount of time.

1 knot = 1 nautical mile per hour = 6076 feet per hour
1 mph =1 mile per hour = 5280 feet per hour

For example, if a train is moving at 50 mph on a track, how would you represent this speed in knots (even though trains are not usually represented in knots)?

To do this problem easily, one must convert the speed in miles per hour that the train is moving to the speed in feet per hour. This is accomplished by multiplying by the number of feet in a mile.
That is,

Now, convert the feet per hour to knots by multiplying by the knots conversion factor (1 knot/ 6076 [feet/hr]).

Exercises:

1. Complete the following chart:

   
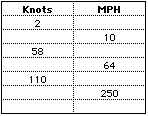
   (answer)

In aerodynamics, speed is also measured by the Mach number, which is the ratio of the speed of the object to the speed of sound. Mach 1 means that you are traveling at the speed of sound or 661.7 knots, or

How would you determine the speed of sound in mph?
Since

and

We can say that Mach 1 = 761.5 mph at sea level.

Since the speed of sound varies with the density of air ( or whatever material it is transmitted through), one needs to determine the density of the air the aircraft is flying through. To compute this, we will use the chart shown below, called the International Civil Aviation Organization Table, or I.C.A.O.

Notice as the altitude increased, the density of air decreases as does the speed of sound in knots.

2. Using the I.C.A.O. table below, complete the following chart:

   
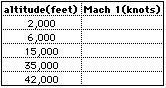
   (answer)

    

3. Now complete the following chart:

   A text only version of this problem and answer are also available.

   
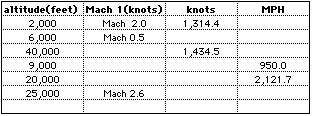
   (answer)