Most modern passenger and military aircraft are powered by
gas turbine engines, which are also called
jet engines. There are several different types
of jet engines, but all jet engines have some partsin common.
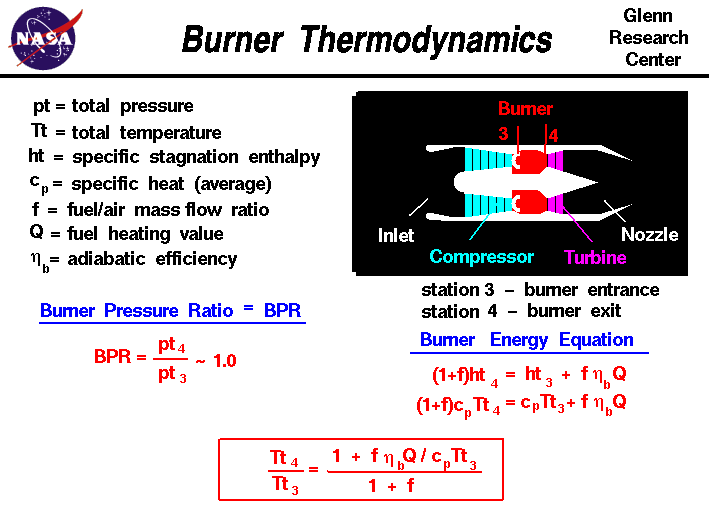
All jet engines have a combustor or
burner in which the air and fuel are mixed and burned. The burning
occurs at a higher pressure than free stream because of the action of
the compressor. The pressure in the
burner remains nearly constant during burning, decreasing by only 1
to 2 per cent. Using our station
numbering, the burner pressure ratio BPR is equal to pt4
divided by pt3 and is nearly equal to one:
BPR = pt4 / pt3 = 1.0
As opposed to the compressor and power
turbine, we cannot simply relate the
total temperature ratio in the burner to the total pressure ratio because the
physical processes are different. In the compressor and turbine, no
heat enters the domain. Under those
adiabatic conditions
the pressure ratio and
temperature ratio are related. In the burner, heat
is released in the combustion process, and the
energy equation
must be used to determine the temperature change. The
energy equation is given by:
(1 + f) * ht4 = ht3 + f * nb * Q
where ht is the specific total enthalpy,
f is the fuel to air mass flow ratio,
Q is the heat release, and nb is an efficiency factor.
The heat release Q depends on the particular fuel that
is being burned and is determined experimentally. An efficiency
factor is applied, as well, to account for losses during burning.
The enthalpy is equal to the
specific heat coefficient
at constant pressure
cp times the temperature which leads to:
(1 + f) * cp * Tt4 = cp * Tt3 + f * nb * Q
With a little algebra, this energy equation can be solved for the temperature
ratio across the burner:
Tt4 / Tt3 = (1 + f * nb * Q / (cp * Tt3) ) / ( 1 + f)
The burner entrance temperature Tt3 is determined by the compressor and
the external flow conditions. The fuel heating value Q is a property of
the particular fuel being used, and the specific heat coefficient cp is
a known property of air. In engine operation, we can set the
fuel flow rate
which determines a value for the fuel/air ratio f and sets the
temperature ratio in the burner.
The burner temperature ratio and pressure ratio determine a value for the
engine temperature ratio, ETR, and
engine pressure ratio, EPR,
which in turn determine the theoretical engine
thrust.
It would appear that we can make the temperature ratio and resulting thrust
as large as we want
by just increasing the fuel flow rate and the fuel/air ratio. However, the
details of the combustion process sets some limits on values of the fuel/air
ratio. And in engine operation, there is a
maximum burner exit temperature Tt4 which is determined by
material limits.
If we try to run the engine hotter than this
maximum temperature, the burner and the turbine will be damaged.
You can now use
EngineSim
to study the effects
of different materials on engine operation.
Activities:

Guided Tours
-
 EngineSim - Engine Simulator:
EngineSim - Engine Simulator:

-
 Burner:
Burner:

-
 Calculating Fuel Flow Rate:
Calculating Fuel Flow Rate:

-
 Combustion:
Combustion:

Navigation ..

- Beginner's Guide Home Page
|
