In the 1700s, Daniel Bernoulli investigated the forces present in a moving fluid. This slide shows one of many forms of Bernoulli's equation. The equation appears in many physics, fluid mechanics, and airplane textbooks. The equation states that the static pressure ps in the flow plus the dynamic pressure, one half of the density r times the velocity V squared, is equal to a constant throughout the flow. We call this constant the total pressure pt of the flow.

As discussed on the gas properties page, there are two ways to look at a fluid; from the large, macro scale properties of the fluid that we can measure, and from the small, micro scale of the molecular motion and interaction. On this page, we will consider Bernoulli's equation from both standpoints.

Macro Scale Derivation

Thermodynamics is the branch of science which describes the macro scale properties of a fluid. One of the principle results of the study of thermodynamics is the conservation of energy; within a system, energy is neither created nor destroyed but may be converted from one form to another. We shall derive Bernoulli's equation by starting with the conservation of energy equation. The most general form for the conservation of energy is given on the Navier-Stokes equation page. This formula includes the effects of unsteady flows and viscous interactions. Assuming a steady, inviscid flow we have a simplified conservation of energy equation in terms of the enthalpy of the fluid:

where ht is the total enthalpy of the fluid, q is the heat transfer into the fluid, and wsh is the useful work done by the fluid.

Assuming no heat transfer into the fluid, and no work done by the fluid, we have:

From the definition of total enthalpy:

where e is the internal energy, p is the pressure, v is the specific volume, and V is the velocity of the fluid. From the first law of thermodynamics if there is no work and no heat transfer, the internal energy remains the same:

The specific volume is the inverse of the fluid density r:

Assuming that the flow is incompressible, the density is a constant. Multiplying the energy equation by the constant density:

This is the simplest form of Bernoulli's equation and the one most often quoted in textbooks. If we make different assumptions in the derivation, we can derive other forms of the equation.

It is important when applying any equation that you are aware of the restrictions on its use; the restrictions usually arise in the derivation of the equation when certain simplifying assumptions about the nature of the problem are made. If you ignore the restrictions, you may often get an incorrect "answer" from the equation. For instance, this form of the equation was derived while assuming that the flow was incompressible, which means that the speed of the flow is much less than the speed of sound. If you use this form for a supersonic flow, the answer will be wrong.

Molecular Scale Derivation

We can make another interpretation of the equation by considering the motion of the gas molecules. The molecules within a fluid are in constant random motion and collide with each other and with the walls of an object in the fluid. The motion of the molecules gives the molecules a linear momentum and the fluid pressure is a measure of this momentum. If a gas is at rest, all of the motion of the molecules is random and the pressure that we detect is the total pressure of the gas. If the gas is set in motion or flows, some of the random components of velocity are changed in favor of the directed motion. We call the directed motion "ordered," as opposed to the disordered random motion.

We can associate a "pressure" with the momentum of the ordered motion of the gas. We call this pressure the dynamic pressure. The remaining random motion of the molecules still produces a pressure called the static pressure. At the molecular level, there is no distinction between random and ordered motion. Each molecule has a velocity in some direction until it collides with another molecule and the velocity is changed. But when you sum up all the velocities of all the molecules you will detect the ordered motion. From a conservation of energy and momentum, the static pressure plus the dynamic pressure is equal to the original total pressure in a flow (assuming we do not add or subtract energy in the flow). The form of the dynamic pressure is the density times the square of the velocity divided by two.

Applications of Bernoulli's Equation

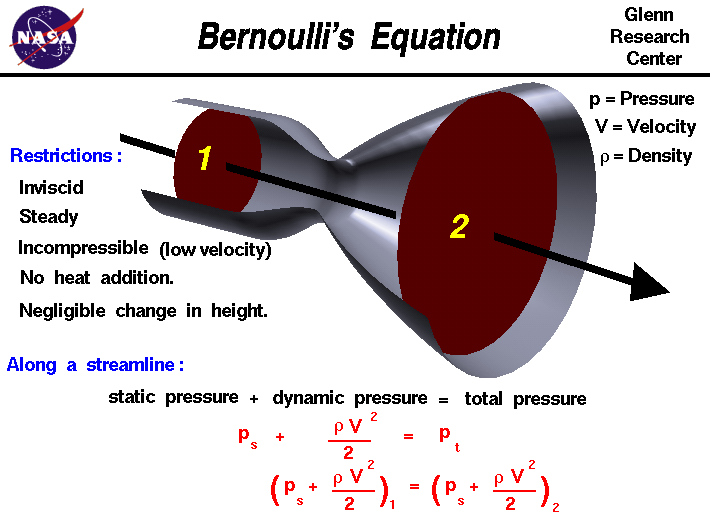
The fluids problem shown on this slide is low speed flow through a tube with changing cross-sectional area. For a streamline along the center of the tube, the velocity decreases from station one to two. Bernoulli's equation describes the relation between velocity, density, and pressure for this flow problem. Since density is a constant for a low speed problem, the equation at the bottom of the slide relates the pressure and velocity at station two to the conditions at station one.

Along a low speed airfoil, the flow is incompressible and the density remains a constant. Bernoulli's equation then reduces to a simple relation between velocity and static pressure. The surface of the airfoil is a streamline. Since the velocity varies along the streamline, Bernoulli's equation can be used to compute the change in pressure. The static pressure integrated along the entire surface of the airfoil gives the total aerodynamic force on the foil. This force can be broken down into the lift and drag of the airfoil.

Bernoulli's equation is also used on aircraft to provide a speedometer called a pitot-static tube. A pressure is quite easy to measure with a mechanical device. In a pitot-static tube, we measure the static and total pressure and can then use Bernoulli's equation to compute the velocity.

• Basic Fluid Dynamics Equations:
• Pitot-Static Tube:

Navigation ..

Beginner's Guide Home Page